
I guess pretty much all of us know that December 21st this year marked the winter solstice, and so – in the northern hemisphere – the shortest day and longest night of the year. But comparatively few people seem to know that this day is not the one when the sun rises latest and sets earliest. The exact dates of those events are, at the latitude of London, just over a week different from the solstice. Specifically, the latest sunrise this year is not until January 1st 2017, and the earliest sunset was on December 12th.
It turns out that the times when the sun rises and sets are governed by a moderately complicated algorithm called the Equation of Time. This obviously varies with your latitude and longitude, but also takes into account the small differences between the solar day and the sidereal day (the day length as measured against the distant and essentially fixed stars), seasonal variations in the earth’s distance from the sun, the apparent size of the solar disk, and a host of other relevant pieces of information. Strictly speaking, one’s height above sea level, and the details of the surrounding terrain also make a difference, but not in a way that’s easy to quantify here. Finally, there are several different definitions of what angle counts as the zenith line, and I have taken the civil definition as opposed to nautical or astronomical.
Once upon a time the calculations would have taken a very long time and lots of paper, but nowadays we can throw the calculation steps into Excel and find out the information for anywhere we want, and for a reasonably long span of time into the past or future. For the curious, a step by step description can be found at this link.
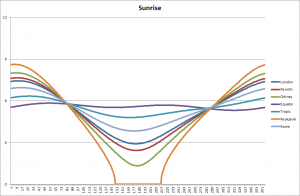
Out of curiosity, I plotted the changes for a series of latitudes from that of Reykjavik in Iceland (just over 64 degrees north) via Orkney, Penrith and London in the UK, through Rome and the Tropic of Cancer to the Equator. For simplicity I just took everything on the zero longitude line (through Greenwich) since I was only interested in changes in latitude. If you wanted to do this for yourself then you would need to adjust for your actual longitude east or west from Greenwich, and your official time zone.
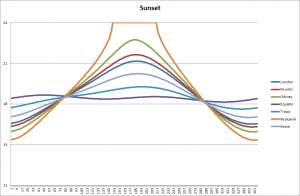
Here’s the corresponding chart for sunset.
A few things stand out at a quick glance. First, the time of sunrise varies considerably at some times of the year even between London and the north of Scotland. Secondly, you don’t have to go all that far north to get to the ‘land of the midnight sun‘. Thirdly, the total range of variation of sunrise is very small at the equator – about 1/2 an hour, as compared with London’s 4 1/2 hours, or Iceland’s 8 1/2 hours. The places where all these lines cross over is at the spring and autumn equinoxes, where night and day are each 12 hours long across the whole globe.
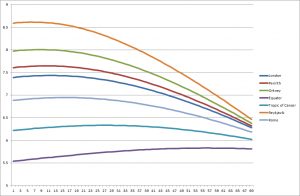
Going back to where we started, and looking carefully at the early part of the year, you can see that the day of latest sunrise happens after the solstice. The further north you go, the closer the two days are together. So in Reykjavik the latest sunrise is on December 26th. Come down to Orkney and it’s the 28th. In London you have to wait until January 1st. In Rome, January 5th. If you lived on the Tropic of Cancer (say in parts of the Sahara, roughly on a level with Kolkata, India) you’d be waiting for the 8th.
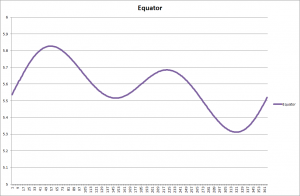
If you live right on the Equator something else comes into play. You get not just a simple days-get-longer then days-get-shorter cycle. Instead there is a more complex curve. Something similar happens in the whole belt of the tropics. This is because there are times when the sun at noon is to the south (as always happens in the northern hemisphere north of the Tropic of Cancer(, but then times when the noonday sun passes overhead and is, for a while, to the north. As it swings over and past you, the day length lengthens and then shortens again – as you can see in the graph.
OK, that’s enough of the Equation of Time for this week. Next time – another oddity about solar movements through the year, together with some thoughts about what this all means for us humans as we have observed the sun through the years. I am convinced that our remote ancestors knew about these patterns (though probably didn’t dress them up in the sines and cosines used by modern maths) and incorporated this knowledge into their monuments and observatories. But more of that next time…

